Demonstration of permutationally invariant quantum tomography
17.12.2010
Multi-partite entangled quantum states play an important role for many quantum information tasks. Especially for an increasing number of particles efficient measurement schemes to characterize these states are needed. In the following publication we profit from the fact, that the most prominent quantum states are permutationally invariant, like for example, the Greenberger-Horne-Zeilinger state and all Dicke states, which include also the W state. Restricting to permutationally invariant quantum states, the measurement effort reduces from exponential to quadratic scaling with the number of qubits.
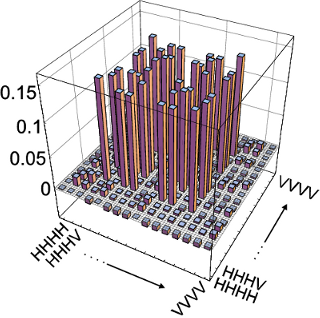
This is demonstrated by a proof of principle experiment for a photonic four qubit symmetric Dicke state. Here, instead of 81 basis settings for full tomography only 15 basis settings have to be measured to reconstruct the permutationally invariant quantum state. In the experiment we achieve an agreement of 94.7% between full and permutationally invariant quantum state tomography demonstrating the applicability of our method. This paves the way for future characterization of permutationally invariant quantum states of many qubits in various physical systems.